Quantum Chromodynamics(QCD) predicts the existence of quark states beyond mesons and baryons, where the number of quarks can exceed three, e.g., multiquark states, multibaryons, nuggets and compact stars made of quark matter. However, due to its nonperturbative nature and infamous sign problem in lattice simulations, currently we have to rely on various QCD inspired effective models to unveil the properties of those objects. For example, the multibaryons were investigated with the bag model^[1-7], nonrelativistic quark cluster model^[8-11], Skyrme model^[12-15], and diquark model^[16]. The interaction among multibaryons with strangeness, i.e., strangeons, were investigated with quark cluster model^[17], extended $ \mathrm{\sigma} $-$ \omega $-$ \rho $ mean-field model^[18], Lennard-Jones potential model^[19], linked bag model^[20-21], and so on. The properties of quark matter were examined in the framework of bag model^[22-23], Nambu-Jona-Lasinio (NJL) model^[24], perturbation model^[25], field correlator method^[26], quasiparticle model^[27-32], equivparticle model^[33-37], etc.

These models, to some extent, have large ambiguities on their predictions of exotic quark states as the model parameters and assumptions are not very well constrained. For example, the H-dibaryons were speculated to be a stable state in the bag model^[1-2], while recent lattice QCD simulations suggests H-dibaryons are weakly bound or unbound^[38-39]. In a large parameter space in models such as bag model, it was shown that strange quark matter (SQM) comprised of approximately equal numbers of u, d, and s quarks are more stable than nuclear matter^[40-43], which permits the existence of strangelets^{[23, 44-46]}, nuclear-ites^[47-48], meteorlike compact ultradense objects^[49], and strange stars^[50-52]. However, in models with spontaneous chiral symmetry breaking, SQM is unstable^[53-54], while the nonstrange quark matter ($ \mathrm{ud}$QM) could be the true ground state^[55]. In such cases, there may be $ \mathrm{ud}$QM nuggets^[55-57] and nonstrange quark stars^[58-63].

Due to the large uncertainties in model predictions and their significant implications, it is essential to constrain the model parameters according to various experimental and observational data. The purpose of our current study is thus to constrain the model parameters of equivparticle model according to the experimental mass spectra of light baryons with spins $ J = 1/2 $ and 3/2. For those consistent with the experimental baryon masses, distinctive correlations between the model parameters are identified. The color-magnetic part of one-gluon-exchange interaction was shown to play an important role on the mass spectra of light baryons, which becomes weaker for baryons with strangeness. The model parameters fixed here can be readily applied to the investigation of exotic states such as $\mathrm{ud}$QM nuggets, strangelets, and compact stars.

The paper is organized as follows. In Sec. 2 we present the theoretical framework of the equivparticle model, where the properties of baryons are obtained in mean field approximations with quarks occupying the $ 1s_{1/2}^{} $ orbits. The numerical results that best reproduce the baryon spectra are then presented in Sec. 3, where the correlations of the confinement strength $ D $, the strong coupling constant $ \alpha_{\rm{s}}^{} $, and quark mass factor $ f $ with respect to the perturbative strength $ C $ are identified and indicated with analytical formulae. We draw our conclusion in Sec. 4.

In the framework of equivparticle model^{[33-37, 64-79]}, the strong interactions among quarks are accounted for with density and/or temperature dependent quark masses, where the mass of quark $ i $ is fixed with

Here $ n_j^{} $ represents the number density for quark flavor $ j $, $ T $ the temperature, and $ m_\mathrm{u0}^{} = 2.16 $ MeV, $ m_\mathrm{d0}^{} = 4.67 $ MeV, $ m_\mathrm{s0}^{} = 93 $ MeV the current masses of quarks^[80-81]. To reflect the effects of quark confinement as in bag model, the density dependent quark masses for zero temperature cases are parametrized as^{[33, 35]}

where $ B $ is the bag constant and $ n_\mathrm{b} = \sum\nolimits_{i = \mathrm{u,d,s}}^{}n_i^{}/3 $ the baryon number density. Alternatively, a cubic-root scaling is derive from the linear confinement and leading-order in-medium chiral condensate^[64], i.e.,

where $ D = -3(2/\pi)^{1/3}\sigma n^*/\sum\nolimits_\mathrm{q}^{}\langle\mathrm{\bar{q}q}\rangle_0$ represents the confinement strength with $ \sigma $ being the string tension, $ n^*\approx0.49 $ ${\rm fm}^{-3}$ the chiral restoration density in the linear expression^[82], and $ \sum\nolimits_\mathrm{q}^{}\langle\mathrm{\bar{q}q}\rangle_0^{} $ the sum of vacuum chiral condensates. Further consideration of one-gluon-exchange interaction suggests^[71]

while $ C = -4(2/\pi)^{1/3}\alpha_{\rm{s}}^{} n^*/\sum\nolimits_\mathrm{q}^{}\langle\mathrm{\bar{q}q}\rangle_0^{} $ varies with the strong coupling constant $ \alpha_{\rm{s}}^{} $. Incorporating the leading-order perturbative interactions at ultrahigh densities, we have found^[75]

where the perturbative strength $ C \approx \pi^{2/3} \sqrt{\frac{2\alpha_{\rm{s}}^{}}{3\pi}} $. An isospin dependent term was also introduced to examine the impacts of quark matter symmetry energy, which was given by

with $ \tau_i^{} $ being the third component of isospin for quark flavor $ i $ and $ \delta = 3(n_\mathrm{d}^{}-n_\mathrm{u}^{})/(n_\mathrm{d}^{}+n_\mathrm{u}^{}) $ the isospin asymmetry^[76]. Recently, we have proposed a similar mass scaling^[57]

where $ C_I^{} $ corresponds to the strength of symmetry energy and is sensitive to the strong interactions among quarks^{[62-63, 76, 83-86]}.

The Lagrangian density of the equivparticle model is determined by

where $ \psi_i^{} $ represents the Dirac spinor of quark flavor $ i $, $ m_i^{} $ the mass, $ q_i^{} $ the charge ($ q_\mathrm{u}^{} = 2e/3 $ and $ q_\mathrm{d}^{} = q_\mathrm{s}^{} = -e/3 $), and $ A_\mu ^{}$ the photon field with the field tensor

The strong interactions are considered with density-dependent quark masses in equivparticle model, where the quark mass in Eq. (8) varies with baryon number density, i.e.,

Note that we have considered both the linear confinement and leading-order perturbative interactions as in Eq. (5), while the current quark masses $ {m}_{i0}^{} $ are multiplied by a factor $ f $ to account for the running quark masses.

Adopting the Euler-Lagrange equation, one obtains the equation of motion for quarks

The term $ V_V^{} $ arises from the density dependent quark masses, i.e.,

The equation of motion for photons is given by

For a spherically symmetric system, the Dirac spinor of quarks can be expanded as

with $ G_{n\kappa}^{}(r)/r $ and $ F_{n\kappa}^{}(r)/r $ being the radial wave functions for the upper and lower components, while $Y_{jm}^l(\theta,\,\phi)$ is the spinor spherical harmonics, i.e.,

The quantum number $ \kappa $ is defined by the angular momenta $(l,\,j)$ as $ \kappa = (-1)^{j+l+1/2}(j+1/2) $. The Dirac equation for the radial wave functions in mean-field approximation is then fixed by

with the single particle energy $ \varepsilon_{n\kappa}^{} $ and the mean-field vector potential

For given radial wave functions, the scalar and vector densities of quarks can be determined by

where the quark numbers $ N_i^{}\ (i = \mathrm{u,\,d,\,s}) $ are obtained by integrating the density $ n_i^{}(r) $ in coordinate space as

The energy of the system can be obtained with

For given $ C $, $ D $ and $ f $, we solve the Dirac Eq. (16), mean field potential Eq. (17), and densities Eq. (18) inside a box by iteration in coordinate space with the grid width $ 0.001 $ fm. The box size varies with quark numbers and is fixed at vanishing densities.

The obtained solution for a baryon can be viewed as a wave packet of the physical particle with various total momentum $\boldsymbol{P}$, where the actual mass $ M_0^{} $ can be approximately obtained with^[87]

The energy $ E_0^{} $ is determined by Eq. (20). And the expectation value of the squared total momentum $ \langle P^2 \rangle $ is obtained as in Ref. [88]. Then the center-of-mass corrections are introduced via the parameter $ \varGamma $ with

Finally, the mean-square radius and mass are obtained with

where $ M_0^{} $ stands for the mass of the corresponding baryon and $ Q $ the charge number.

The one-gluon-exchange interaction is treated as a correction to the mass of Eq. (24), which is obtained with

According to the quark wave functions in Eq. (14), the color current of quark $ i $ is given by

where

The one-gluon-exchange interaction can be divided into the color-electric and -magnetic parts. To simplify our calculation, we neglect the self-energy diagram and the color-electric part of one-gluon-exchange interaction, which are expected to be included in the quark mass scaling^[71]. For the cases with $ l = 0 $ (occupying the $ 1s_{1/2}^{} $ state), we have $|Y_{jm}^l(\theta,\,\phi)|^2 = 1/4\pi$. Then Eq. (25) for baryons is finally reduced to

The interaction coefficients from quark spins are fixed with

where $ S_{i, \,j}^{} = 0 $ or 1 is the total spin of the pair of quarks $ i $ and $ j $. The total baryon mass is finally obtained by adding the corrections to Eq. (24), i.e.,

Based on the formulae introduced in Sec. 2, the baryon masses are then fixed by Eq. (32), which include both the center-of-mass corrections in Eq. (24) and color-magnetic part of one-gluon-exchange interactions in Eq. (30). We then fit to the mass spectra of baryons with angular momentum $ l = 0 $ and masses $ M<1.35 $ GeV at $ C = -1 $, $ -0.5 $, $ -0.2 $, 0, 0.2, 0.5, 0.7, 1, where the experimental baryon masses are taken from Particle Data Group^[81]. The obtained parameter sets that best reproduce the experimental values are indicated in Table 1.

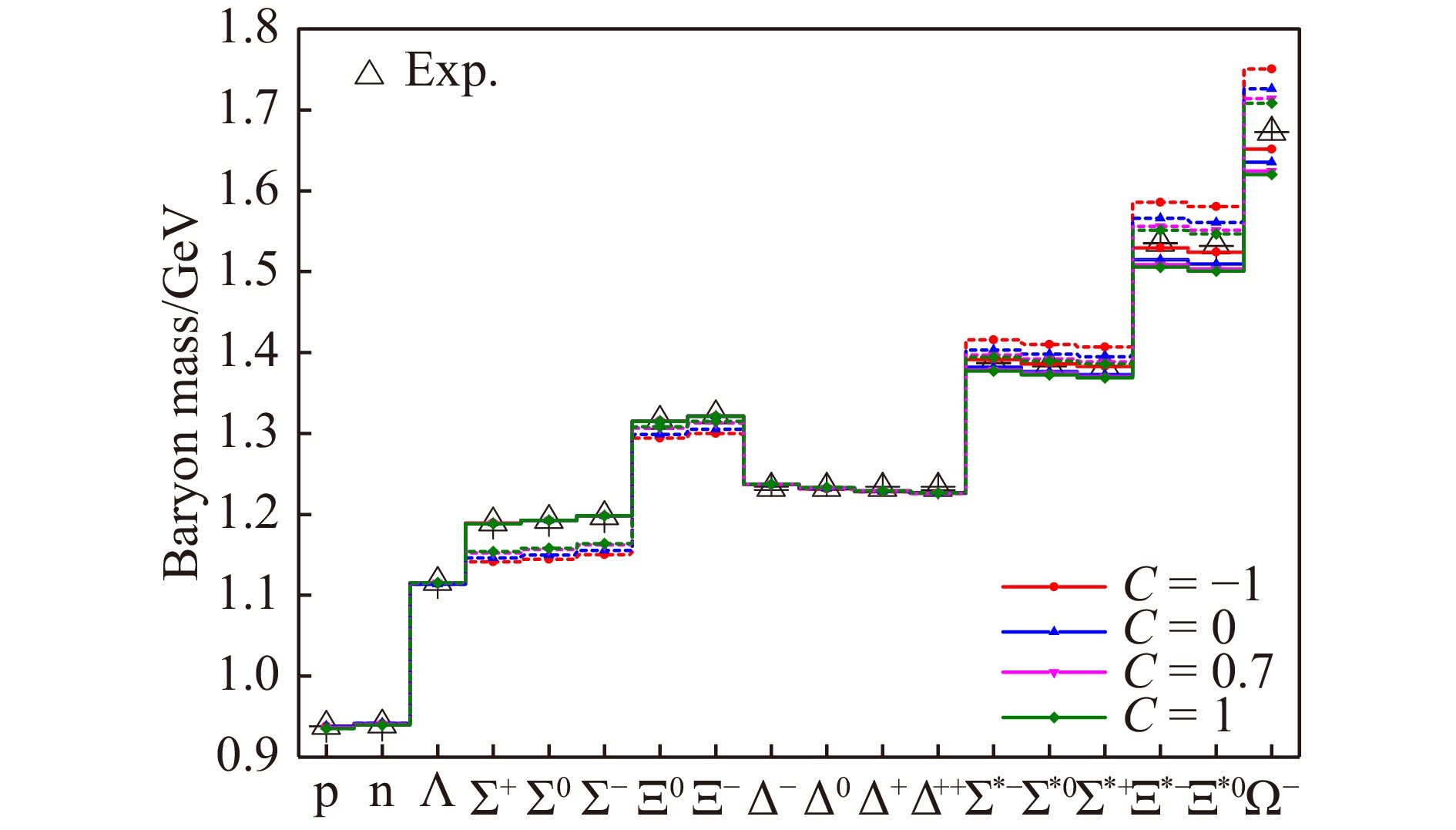
To illustrate our results, in Fig. 1 we present the obtained baryon masses by taking $ C = -1 $, 0, 0.7, and 1, where the corresponding parameter sets are listed in Table 1. In particular, we first fix the parameters $ D $, $ f $, and $ \alpha_\mathrm{s}^\mathrm{ll} $ ($\mathrm{l = u, d }$) by reproducing the masses of $\mathrm{p}$, $\mathrm{n}$, $ \Lambda $, and $ \Delta $. According to the quark mass scaling in Eq. (10), the confinement ($ D $) and perturbative ($ C $) interactions provide the bulk properties of baryon mass spectra, where a distinctive $ C $-$ D $ correlation can be obtained. The fine structures such as the mass differences between nucleons $\mathrm{N} $ ($\mathrm{p}$, $\mathrm{n}$) and $ \Lambda $-hyperons $ \varDelta M_\mathrm{N\Lambda}^{} $ determines $ f $, while $ \varDelta M_\mathrm{N\Delta}^{} $ determines the strong coupling constant $ \alpha_\mathrm{s}^\mathrm{ll} $ for the color-magnetic part of one-gluon-exchange interaction. Based on those parameter sets and assuming unified strong coupling constants $ \alpha_\mathrm{s}^\mathrm{ss} = \alpha_\mathrm{s}^\mathrm{ls} = \alpha_\mathrm{s}^\mathrm{ll} $, we then estimate the masses of other baryons, which are indicated by the dots connected by dashed lines in Fig. 1. It is evident that our model slightly underestimates the masses of $ \Sigma $, $ \Xi $ and overestimates the masses of their excited states $ \Sigma^* $, $ \Xi^* $ as well as $ \Omega $. The slight deviations from the experimental baryon masses could be attributed to the variation of strong coupling constant on quark masses, which is in principle running with the energy scale.

Figure 1.  The obtained masses of baryons consisting of light quarks adopting the parameter sets indicated in Table 1. The dots connected by dashed lines are fixed by taking unified values for strong coupling constants $ \alpha_\mathrm{s}^\mathrm{ss}=\alpha_\mathrm{s}^\mathrm{ls}=\alpha_\mathrm{s}^\mathrm{ll} $, while those connected by the solid lines (indicated in Table 2) adopt strong coupling constants that vary with the contents of interacting quark pairs.(color online)

To better reproduce the mass spectra of light baryons, we thus adopt different strong coupling constants for quark pairs with strangeness, i.e., $ \alpha_\mathrm{s}^\mathrm{ss}\neq\alpha_\mathrm{s}^\mathrm{ls}\neq\alpha_\mathrm{s}^\mathrm{ll} $. The strong coupling constants are then fixed by fitting $ \alpha_\mathrm{s}^\mathrm{ls} $ to the mass of $ \Sigma^0 $ and $ \alpha_\mathrm{s}^\mathrm{ss} $ to the masses of $ \Xi $, which are indicated in Table 1. Then the mass corrections $ \varDelta M $ from the color-magnetic part of one-gluon-exchange interactions are modified for $ \Sigma $, $ \Xi $, $ \Sigma^* $, $ \Xi^* $ and $ \Omega $. The obtained mass spectra are indicated by the dots connected by the solid curves in Fig. 1, where the agreement between predicted and experimental values is obviously improved. In particular, the experimental values of baryons with $ M<1.5 $ GeV are well reproduced. For more massive baryons $ \Xi^* $ and $ \Omega $, our model slightly underestimates their masses. Nevertheless, the deviation decreases if we adopt negative $ C $, which in principle incorporates the color-electric part of one-gluon-exchange interactions^[71].

The numerical results correspond to the dots connected by the solid curves in Fig. 1 are presented in Table 2. The root-mean-square deviations $ \sigma $ from the experimental values of $ N = 18 $ baryons are indicated, which are fixed by

with $ M_\mathrm{cal}^i $ and $ M_\mathrm{exp}^i $ being the numerical and experimental baryon masses. It is found that $ \sigma $ is generally on the order of $ 10 $ MeV and increase with $ C $. Note that for baryons with spin $ J = {1}/{2} $, quarks form pairs with total spin $ S = 0 $ and 1 as indicated in Table 2, which determines the interaction coefficients $ a_{i,j}^{} $ for the color-magnetic part of one-gluon-exchange interaction in Eq. (31). For baryons with spin $ J = {3}/{2} $, all three quarks' spins are aligned.

In Fig. 2 we present the center-of-mass correction parameter $ \varGamma $, the root-mean-square radius $ \sqrt{\left\langle r^2 \right\rangle} $ and the mass correction $ \varDelta M $ from the color-magnetic part of one-gluon-exchange interaction, which are in correspondence to the baryon spectra indicated in Fig. 1 and Table 2. For baryons obtained with different parameter sets, it is found that the center-of-mass correction parameter $ \varGamma $ is generally inversely proportional to the root-mean-square radius $ \sqrt{\left\langle r^2 \right\rangle} $, which is due to the enhancement of kinetic energy of quarks as baryons become more compact. Nevertheless, the variation of $ \varGamma $ share similar trends as that of $ \sqrt{\left\langle r^2 \right\rangle} $, where the strange quark mass plays the leading role. The mass corrections $ \varDelta M $ of $\mathrm{p}$, $\mathrm{n}$, $ \Lambda $, and $ \Delta $ from the color-magnetic part of one-gluon-exchange interactions are invariant to the adopted parameter set, which is mainly because we fit our parameter sets based on those baryons. If we examine other baryons, as indicated by the dashed lines, slight variations are observed for hyperons when we take $ \alpha_\mathrm{s}^\mathrm{ss} = \alpha_\mathrm{s}^\mathrm{ls} = \alpha_\mathrm{s}^\mathrm{ll} $ (dashed lines). Similar situation is observed if we take $ \alpha_\mathrm{s}^\mathrm{ss}\neq\alpha_\mathrm{s}^\mathrm{ls}\neq\alpha_\mathrm{s}^\mathrm{ll} $, where $ \varDelta M $ varies little for $ \Sigma $ and $ \Xi $ since $ \alpha_\mathrm{s}^\mathrm{ls} $ and $ \alpha_\mathrm{s}^\mathrm{ss} $ are fixed according to their masses. This suggests that the mass correction $ \varDelta M $ from the color-magnetic part of one-gluon-exchange interactions is insensitive to the adopted model parameters, which are expected to play important roles in color superconductivity in dense quark matter^[89], the strength of symmetry energy coefficient $ C_I^{} $ of quark matter as in Eq. (7), as well as the strong interactions among baryons^[90].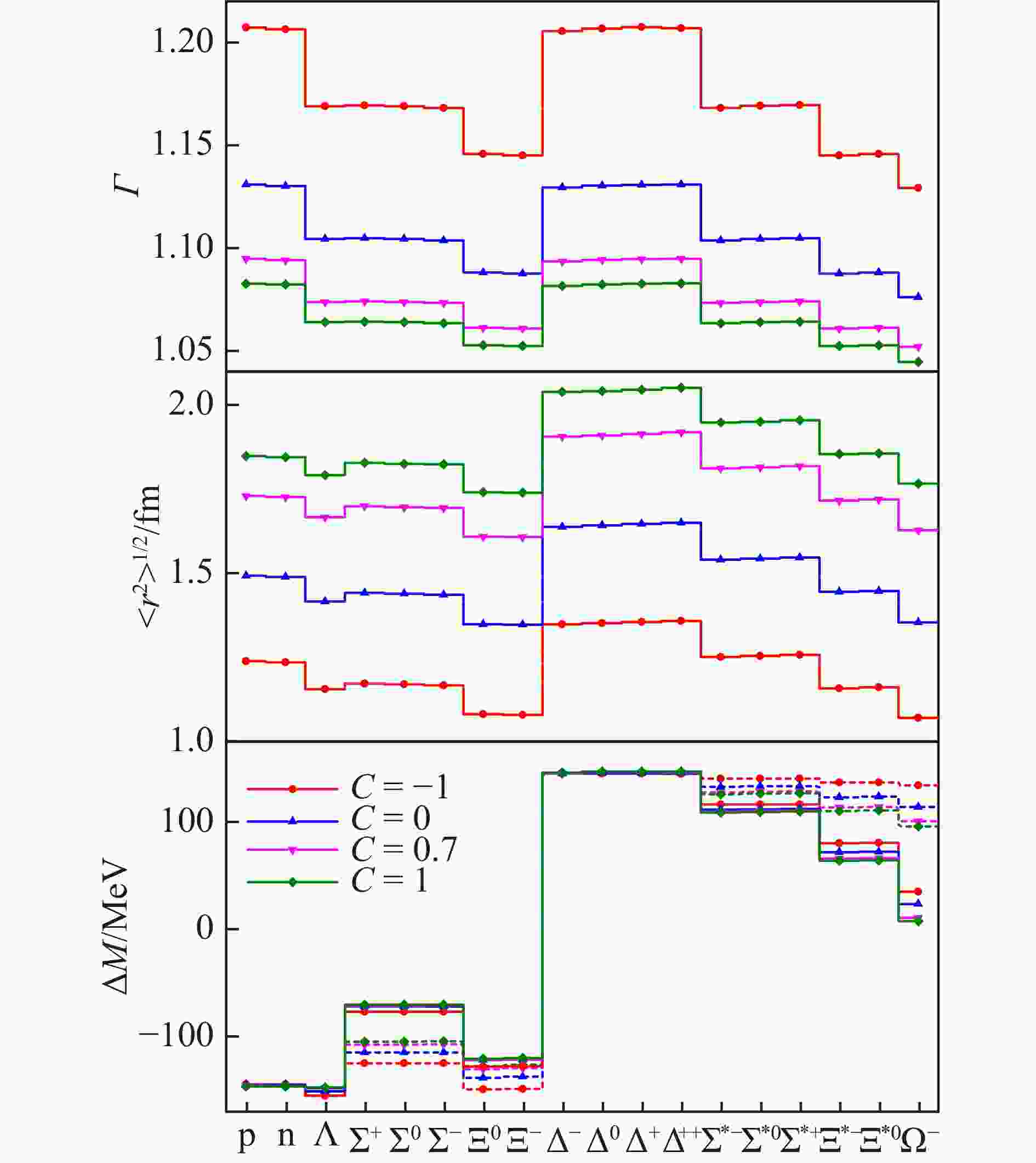

Figure 2.  The center-of-mass correction parameter $\varGamma$, the root-mean-square radius $\sqrt{\left\langle r^2 \right\rangle}$ and the mass correction $\varDelta M$ from the color-magnetic part of one-gluon-exchange interactions obtained with Eqs. (22), (23) and (30). (color online)

As indicated in Table 1, we have considered additional four parameter sets with $ C = -0.5 $, $ -0.2 $, 0.2, and 0.5, where the parameters $ D $, $ f $, $ \alpha_\mathrm{s}^\mathrm{ll} $, $ \alpha_\mathrm{s}^\mathrm{ls} $, and $ \alpha_\mathrm{s}^\mathrm{ss} $ are fixed in a same manner. The parameters that best reproduce the experimental baryon spectra are then plotted in Fig. 3, which can be well approximated by the following formulae, i.e.,

Figure 3.  The fitted parameters for the quark mass scaling in Eq. (10), the strong coupling constants, and mass the root-mean-square deviation $\sigma$ as functions of parameter $C.$(color online)

It is found that the confinement strength $ D $(in MeV²) decreases with the perturbative strength $ C $, which is attributed to the fixed masses of baryons as both confinement and perturbative interactions contribute positively to the total mass. The mass factor $ f $ ranges from 2.19 to 2.79, suggesting the strange quark mass should be at least 210 MeV to account for the mass difference between $\mathrm{N} $ and $ \Lambda $. This has significant implications on the stability of strange quark matter, where $\mathrm{ud} $ quark matter might be more stable^[55]. Meanwhile, we note $ f $ decreases slightly with $C$, where the mass difference $ \varDelta M_\mathrm{N\Lambda}^{} $ is mainly from $ fm_\mathrm{s0}^{} $ instead of its kinetic energy as the baryons become less compact. In this work, we have considered two scenarios for the strong coupling constants of one-gluon-exchange interactions, i.e., a unified value with $ \alpha_\mathrm{s}^{} = \alpha_\mathrm{s}^\mathrm{ss} = \alpha_\mathrm{s}^\mathrm{ls} = \alpha_\mathrm{s}^\mathrm{ll} $ and flavor-dependent ones with $ \alpha_\mathrm{s}^\mathrm{ss} \neq \alpha_\mathrm{s}^\mathrm{ls} \neq \alpha_\mathrm{s}^\mathrm{ll} $. To better describe the masses of hyperons, it is found that $ \alpha_\mathrm{s}^\mathrm{ss} < \alpha_\mathrm{s}^\mathrm{ls} < \alpha_\mathrm{s}^\mathrm{ll} $, which is reasonable as $ \alpha_\mathrm{s}^{} $ should decrease with the total mass of each quark pair. Meanwhile, the strong coupling constants generally increase with $ C $, which may be attributed to the running coupling constant with respect to the density or radius, e.g., those in Ref. [91].

The relative differences between our calculation and the experimental baryon masses are indicated in Fig. 4, where the strong coupling constant are flavor-dependent. The root-mean-square deviations for the cases with unified strong coupling constant, flavor-dependent strong coupling constant and light baryons with $ M<1.35 $ GeV are indicated in the lower panel of Fig. 3, which are obtained with Eq. (33) at $ N = 18 $, 18 and 12, respectively. As $ C $ increases, it is found that $ \sigma $ decreases if unified strong coupling constant is adopted. Meanwhile, as indicated in Fig. 4, the deviations from the experiment baryon masses increases with $ C $ for flavor-dependent strong coupling constant, i.e., $ \sigma $ increases with $ C $. Since we have fitted our parameters according to the masses of $\mathrm{p}$, $\mathrm{n} $, $ \Lambda $, $ \Delta $, $ \Sigma $ and $ \Xi $, the relative deviations are much smaller for light baryons with $ M<1.35 $ GeV in comparison to heavier ones. Then the root-mean-square deviation $ \sigma\approx2.5 $-3.9 MeV and is insensitive to the parameter sets adopted here. In such cases, we expect the model parameters indicated in Table 1 are best applied to systems with small baryon chemical potentials $ \mu_\mathrm{b}^{}\lesssim 1.35 $ GeV.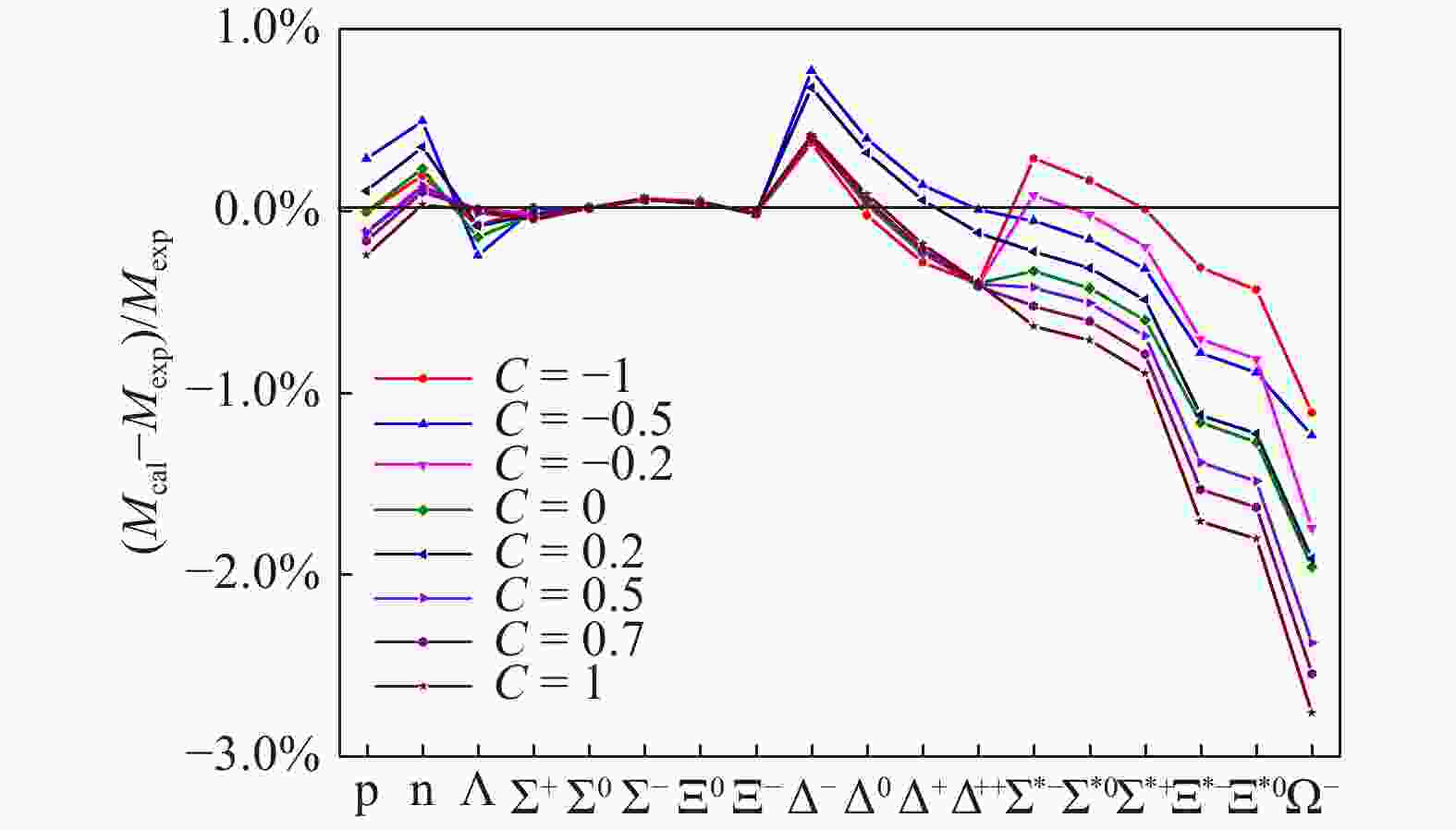

Figure 4.  The relative differences between the obtained masses of baryons and their experimental values. The baryons are all comprised of light quarks with masses $M_\mathrm{exp}^{}<1.7$ GeV. (color online)

In Table 3 we present the mass gaps of baryons in various isospin-multiplets, which indicate the isospin breaking effects in baryons and are fixed by $M(I,\,I_3^{}-1)-M(I,\,I_3^{})$ or $[M(I,\,I_3^{}-2)-M(I,\,I_3^{})]/2$. The corresponding quark mass splitting is obtained with $ \varDelta m_\mathrm{ud}^{} = f (m_\mathrm{d0}^{}-m_\mathrm{u0}^{}) $, where the mass factor $ f $ indicated in Table 1 is adopted. Evidently, the mass gaps in isospin-multiplets are generally small comparing with baryon masses, which is attributed to the small mass differences $ \varDelta m_\mathrm{ud}^{} $ between $\mathrm{u} $ and $\mathrm{d}$ quarks. The mass gaps are dominated by $ \varDelta m_\mathrm{ud}^{} $, which are slightly dampened due to the nonzero kinetic energy of quarks. The Coulomb interaction contributes ~0.5-1 MeV to the masses of baryons with charge number $ Q = \pm1 $, which reduces the mass splitting for nucleons $\mathrm{N} $ but increases for $ \Xi $ and $ \Xi^*. $ The one-gluon-exchange interaction usually works against the isospin breaking effects except for $ \Xi $, e.g., the corresponding contributions to the mass splittings for $\mathrm{N} $, $ \Sigma $, $ \Xi $, $ \Delta $, $ \Sigma^* $ and $ \Xi^* $ are $ -0.17 $, $ -0.02 $, 0.67, $ -0.17 $, $ -0.31 $ and $ -0.34 $ MeV at $ C = 0 $, respectively. Comparing with the experimental values, our model slightly overestimates the mass gaps for $\mathrm{N} $, $ \Sigma $, $ \Sigma^* $, $ \Xi^* $ and underestimates that of $ \Xi $. This could in principle be improved by reducing $ \varDelta m_\mathrm{ud} ^{} $. In general, the mass gaps obtained in our equivparticle model vary little with respect to the adopted parameter sets and deviate slightly from the experimental values ($ \lesssim3 $ MeV).

In this work we fix the parameter sets of equivparticle model including both confinement and leading-order perturbative interactions, which are attained by fitting to the mass spectra of baryons. Distinctive correlations of the confinement strength $ D $, the strong coupling constant $ \alpha_{\rm{s}}^{} $, and the quark mass factor $ f $ with respect to the perturbative strength $ C $ are identified and can be well approximated by analytical formulae. Meanwhile, we have found that the color-magnetic part of one-gluon-exchange interaction is essential to explain the mass gap between baryons with spins $ J = 1/2 $ and 3/2, which reaches up to 300 MeV. Two sets of strong coupling constants for the one-gluon-exchange interaction between quarks are considered in this work, i.e., unified and flavor-dependent ones. If larger perturbative strength $ C $ is adopted, the deviation of model calculations from experimental baryon masses increases for unified strong coupling constants and decreases for flavor-dependent ones. Based on the parameters fixed in this work, the equivparticle model gives good prediction on the masses of light baryons with $ M<1.35 $ GeV, where the root-mean-square deviation is approximately 3 MeV and insensitive to the adopted parameter sets. We thus expect that the equivparticle model with constrained parameter sets are applicable to $\mathrm{ud} $QM nuggets, strangelets, and quark matter with small baryon chemical potentials $ \mu_\mathrm{b}^{}\lesssim 1.35 $ GeV.

Acknowledgments This work was supported by National SKA Program of China(Grant Nos. 2020SKA-0120300, 2020SKA0120100), National Natural Science Foundation of China(Grant Nos. 12275234, 12105241, 11875052, 12005005 & 12205093), and the Scientific Research Start-up Fund of Talent Introduction of Suqian University under Grant No. Xiao2022XRC061.